|
||||||||||
|
|
Fibonacci Numbers: A Measure of Beauty By Harun Yahya [Allah has appointed a measure for all things.] (Qur’an, 65: 3) The Wikipedia encyclopaedia describes beauty as ‘the phenomenon of the experience of pleasure, through the perception of balance’. Everyone admires beauty in nature and the unique balance found in it. Some say that this balance and perception of beauty is due to the Golden number or the ratio that gives certain things their exquisitness. If a pleasing or exceedingly balanced form is achieved in terms of elements of application or function, it is there that we may look for a function of the Golden Number. The Golden Number is a product not of mathematical imagination, but of a natural principle related to the laws of equilibrium.[1][1] What do the pyramids in Egypt, Leonardo da Vinci’s portrait of the Mona Lisa, sunflowers, the snail, the pine cone and your fingers all have in common? The answer to this question lies hidden in a sequence of numbers discovered by the Italian mathematician Fibonacci. The characteristic of these numbers, known as the Fibonacci numbers, is that each one consists of the sum of the two numbers before it.[1][2]
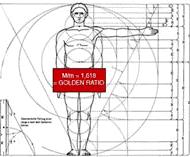
Fibonacci numbers 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1597, 2584, … Fibonacci numbers have an interesting property. When you divide one number in the sequence by the number before it, you obtain numbers very close to one another. In fact, this number is fixed after the 13th number in the series. This number is known as the “golden ratio.” GOLDEN RATIO = 1.618 233 / 144 = 1.618 377 / 233 = 1.618 610 / 377 = 1.618 987 / 610 = 1.618 1597 / 987 = 1.618 2584 / 1597 = 1.618 The Golden Ratio When conducting their researches or setting out their products, artists, scientists and designers take the human body, the proportions of which are set out according to the golden ratio, as their measure. Leonardo da Vinci and Le Corbusier took the human body, proportioned according to the golden ratio, as their measure when producing their designs. The human body, proportioned according to the golden ratio, is taken as the basis also in the Neufert, one of the most important reference books of modern-day architects. The Golden Ratio in the Human Body
· nose · Length of face / distance between tip of jaw and where the eyebrows meet · Length of mouth / width of nose · Width of nose / distance between nostrils · Distance between pupils / distance between eyebrows Golden Proportion in the Lungs In a study carried out between 1985 and 1987,[i][5] the American physicist B. J. West and Dr. A. L. Goldberger revealed the existence of the golden ratio in the structure of the lung. One feature of the network of the bronchi that constitutes the lung is that it is asymmetric. For example, the windpipe divides into two main bronchi, one long (the left) and the other short (the right). This asymmetrical division continues into the subsequent subdivisions of the bronchi.[ii][6] It was determined that in all these divisions the proportion of the short bronchus to the long was always 1/1.618. The Golden Rectangle and the Design in the Spiral
A rectangle, the proportion of whose sides is equal to the golden ratio is known as a “golden rectangle.” A rectangle whose sides are 1.618 and 1 unit long is a golden rectangle. Let us assume a square drawn along the length of the short side of this rectangle and draw a quarter circle between two corners of the square. Then, let us draw a square and a quarter circle on the remaining side and do this for all the remaining rectangles in the main rectangle. When you do this you will end up with a spiral. The British aesthetician William Charlton explains the way that people find the spiral pleasing and have been using it for thousands of years stating that we find spirals pleasing because we are easily able to visually follow them.[iii][7] The spirals based on the golden ratio contain the most incomparable designs you can find in nature. Examples we can give of this are the spiral sequences on the sunflower and the pine cone. The Design in Sea Shells When investigating the shells of mollusks, which live at the bottom of the sea, the form and the structure of the internal and external surfaces of the shells attracted scientists’ attention.
The internal surface is smooth, while the outside surface is fluted. The mollusk’s body is inside the shell. The outside edges of the shell augment its rigidity and, thus, increase its strength. The shell’s form is astonishing in its perfection highlighting the beauty of its creation. The spiral idea in shells is expressed in the perfect geometrical form, in a surprisingly beautiful, “sharpened” design.[i][8] The shells of most mollusks grow in a logarithmic spiral manner. There can be no doubt, of course, that these animals are unaware of even the simplest mathematical calculation, let alone logarithmic spirals. So how is it that the creatures in question can know that this is the best way for them to grow? How do these animals, that some scientists describe as “primitive,” know that this is the ideal form for them? It is impossible for growth of this kind to take place in the absence of a consciousness or intellect. That consciousness exists neither in mollusks nor, despite what some scientists would claim, in nature itself. It is totally irrational to seek to account for such a thing in terms of chance. This design can only be the product of the Almighty Allah.
Growth of this kind was described as “gnomic growth” by the biologist Sir D’Arcy Thompson, an expert on the subject, who stated that it was impossible to imagine a simpler system, during the growth of a seashell, than which was based on widening and extension in line with identical and unchanging proportions. As he pointed out, the shell constantly grows, but its shape remains the same.[i][9] One can see one of the best examples of this type of growth in a nautilus, just a few centimetres in diameter. C. Morrison describes this growth process, which is exceptionally difficult to plan even with human intelligence, stating that along the nautilus shell, an internal spiral extends consisting of a number of chambers with mother-of-pearl (calcium carbonate and conchiolin secreted by the mantle of mollusks) lined walls. As the animal grows, it builds another chamber at the mouth of the spiral shell larger than the one before it, and moves forward into this larger area by closing the door behind it with a layer of mother-of-pearl.[i][10] Growth in a spiral form in the animal world is not restricted to the shells of mollusks. Animals such as antelopes, goats and rams complete their horn development in spiral forms based on the golden ratio.[i][11] The Golden Ratio in the Hearing and Balance Organ The cochlea in the human inner ear serves to transmit sound vibrations. This bony structure, filled with fluid, has a logarithmic spiral shape with a fixed angle of α=73°43´ containing the golden ratio. The Golden Ratio in DNA The molecule in which all the physical features of living things are stored, too, has been created in a form based on the golden ratio. The DNA molecule, the very program of life, is based on the golden ratio. DNA consists of two intertwined perpendicular helixes. The length of the curve in each of these helixes is 34 angstroms and the width 21 angstroms. (1 angstrom is one hundred millionth of a centimetre.) 21 and 34 are two consecutive Fibonacci numbers. The Golden Ratio in Snow Crystals The golden ratio also manifests itself in crystal structures. Most of these are in structures too minute to be seen with the naked eye. Yet you can see the golden ratio in snow flakes. The various long and short variations and protrusions that comprise the snow flake all yield the golden ratio.[i][12] The Golden Ratio in Space In the universe there are many spiral galaxies containing the golden ratio in their structures. The Golden Ratio in Physics You encounter Fibonacci series and the golden ratio in fields that fall under the sphere of physics. When a light is held over two contiguous layers of glass, one part of that light passes through, one part is absorbed, and the rest is reflected. What happens is a “multiple reflection.” The number of paths taken by the ray inside the glass before it emerges again depends on the number of reflections it is subjected to. In conclusion, when we determine the number of rays that re-emerge, we find that they are compatible with the Fibonacci numbers.[i][13] The fact that a great many unconnected animate or inanimate structures in nature are shaped according to a specific mathematical formula is one of the clearest proofs that these have been specially designed. The golden ratio is an aesthetic rule well known and applied by artists. Works of art based on that ratio represent aesthetic perfection. Plants, galaxies, micro-organisms, crystals and living things designed according to this rule imitated by artists are all examples of Allah’s superior artistry. Allah reveals in the Qur’an that He has created all things with a measure. Some of these verses read: [Allah has appointed a measure for all things.] (Qur’an, 65: 3) [Everything has its measure with Him.] (Qur’an, 13: 8) **The author, who writes under the pen-name Harun Yahya, has published many books on political, faith-related and scientific issues. Some of the books of the author have been translated into English, German, French, Spanish, Italian, Portuguese, Albanian, Arabic, Polish, Russian, Bosnian, Indonesian, Turkish, Tatar, Urdu and Malay and published in the countries concerned. Visit his website at www.harunyahya.com or contact him at info@harunyahya.com [1] Mehmet Suat Bergil, Doğada/Bilimde/Sanatta, Altın Oran (The Golden Ratio in Nature/Science/Art), Arkeoloji ve Sanat Yayinlari, 2nd Edition, 1993, p. 155. [2] Guy Murchie, The Seven Mysteries of Life, First Mariner Boks, New York, pp. 58-59. [3] J. Cumming, Nucleus: Architecture and Building Construction, Longman, 1985. [4] Mehmet Suat Bergil, Doğada/Bilimde/Sanatta, Altın Oran (The Golden Ratio in Nature/Science/Art), Arkeoloji ve Sanat Yayinlari, 2nd Edition, 1993, p. 87. [5] A. L. Goldberger, et al., “Bronchial Asymmetry and Fibonacci Scaling.” Experientia, 41 : 1537, 1985. [6] E. R. Weibel, Morphometry of the Human Lung, Academic Press, 1963. [7] William Charlton, Aesthetics: An Introduction, Hutchinson University Library, London, 1970. [8] “The ‘Golden’ spirals and ‘pentagonal’ symmetry in the alive Nature,” online at: http://www.goldenmuseum.com/index_engl.html [9] D’Arcy Wentworth Thompson, On Growth and Form, C.U.P., Cambridge, 1961. [10] C. Morrison, Along The Track, Withcombe and Tombs, Melbourne.
[11] “The ‘Golden’ spirals and ‘pentagonal’ symmetry in the alive Nature,” online at: http://www.goldenmuseum.com/index_engl.html [12] Emre Becer, “Biçimsel Uyumun Matematiksel Kuralı Olarak, Altın Oran” (The Golden Ratio as a Mathematical Rule of Formal Harmony), Bilim ve Teknik Dergisi (Journal of Science and Technology), January 1991, p.16. [13] V.E. Hoggatt, Jr. and Bicknell-Johnson, Fibonacci Quartley, 17:118, 1979. The works posted on this page reflect solely the opinions of the authors.
|
|||||||||